Question: In a single point turning tool, the side rake angle and orthogonal rake angle are equal. φ is the principal cutting edge angle and its range is 0° ≤φ≤ 90°. The chip flows in the orthogonal plane. Determine the value of φ. [GATE 2008]

Answer: Although in majority of the analysis for straight turning, it is assumed that the chip is flowing in orthogonal direction, but actually chip flow direction deviates from orthogonal plane. There are three reasons for such deviation—(i) presence of non-zero inclination angle, (ii) presence of substantially larger nose radius as compared to depth of cut, and (iii) active participation of auxiliary cutting edge in chip formation or material removal. Among these three, the influence of inclination angle cannot be neglected, while others can be neglected as their influence is meagre.

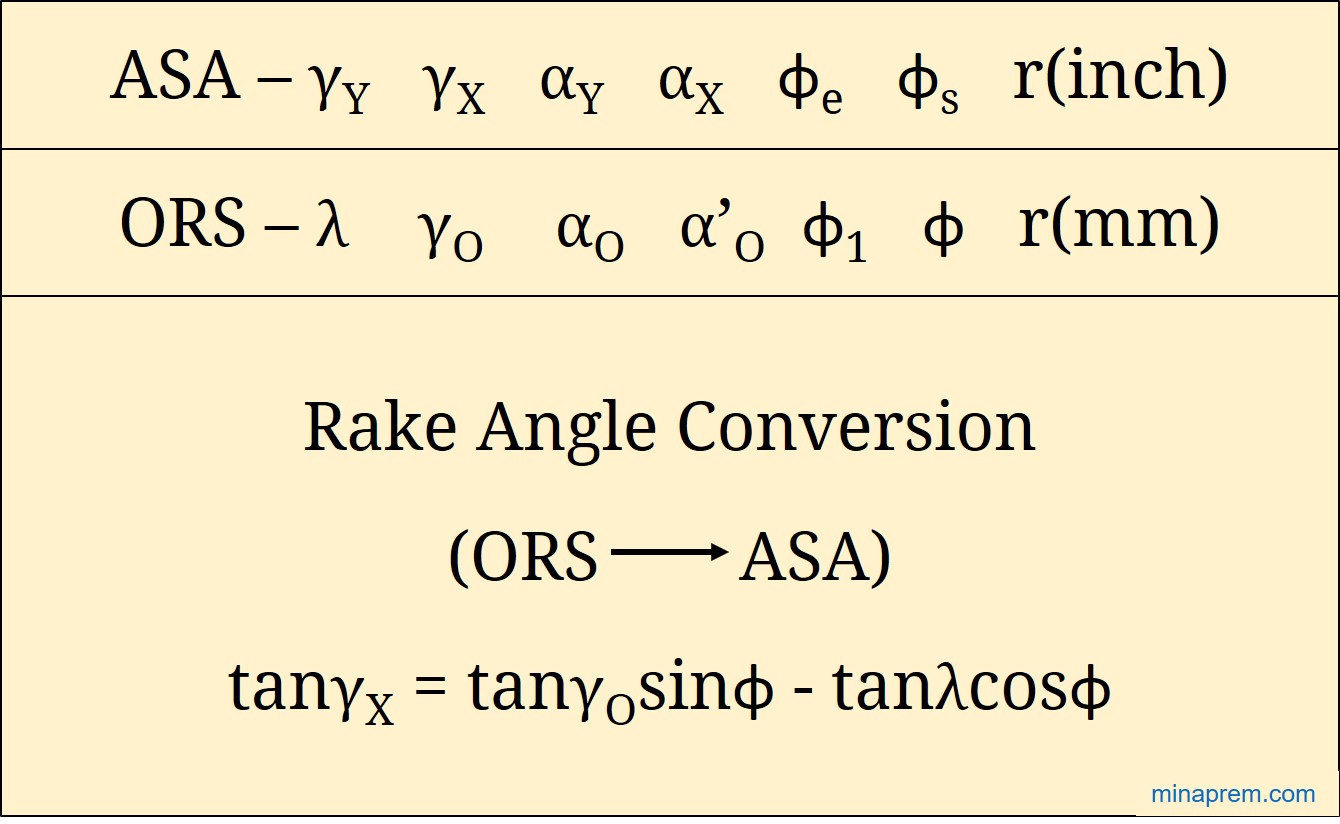
Emphasis is been given in the question that the chip flows in orthogonal direction. Thus it indirectly suggests that the inclination angle (λ) of the cutting tool is zero. Without providing any numerical value, the question also states that side rake angle (γ_X) and orthogonal rake angle (γ_O) are equal. It is to be remembered that the side rake angle (γ_X) is used in ASA (American Standards Association) system of tool designation; while the orthogonal rake angle (γ_O) is used in ORS (Orthogonal Reference System). The inclination angle (λ) is another one element of ORS system.

Now it is the role of tool angle conversion from ORS to ASA. The side rake angle (γ_X) of cutting tool as used in ASA system can be expressed as a function of orthogonal rake angle (γ_O) and inclination angle (λ) of the ORS system. The general formula also include principal cutting edge angle (φ), as provided below.

\[{\gamma _X} = f({\gamma _O},\lambda ,\phi )\]

\[\tan {\gamma _X} = \tan {\gamma _O}\sin \phi  – \tan \lambda \cos \phi \]

Now calculate principal cutting edge angle

In this particular case, as given in question:

side rake angle (γ_X) = orthogonal rake angle (γ_O)

Also, inclination angle (λ) = 0°

Therefore, solution of the above expression leads to:

\(\tan {\gamma _X} = \tan {\gamma _O}\sin \phi  – \tan 0\cos \phi \) [∵ λ=0°]

or, \(\tan {\gamma _X} = \tan {\gamma _O}\sin \phi \)

or, \(\sin \phi  = 1\) [∵ γ_X = γ_O]

or, \(\sin \phi  = \sin 90\) [∵ 0°≤φ≤90°]

or, \(\phi  = 90°\)

Therefore, principal cutting edge angle of the cutting tool is 90°.